Research summary
I work in mechanics, which is, arguably, the oldest branch of applied mathematics. If I had to summarise the essense of my research it would be, perhaps, best to say that I am interested in finding simple, analytical, if necessary — approximate, answers to various problems in solid mechanics. Modern availablity of the relatively cheap computer power means that numerical solutions are often available even for extremely complicated problems of applied physics and engineering. Of course, if one seeks an insight into the qualitative behaviour of the solution, and/or its parametric dependencies, the numerical solutions may be just as difficult to interpret as the original physical phenomena. In my opinion, this means that the job of a contemporary applied mathematician is to find solutions that illuminate, i.e. the solutions that enable an improved qualitative understanding of the studied phenomena, even if the precision of such solutions may suffer as the result.
Below I attempted to identify and describe the most well-developed strands of my reseach. If you have any comments or questions about these (or related) topics, please feel free to drop me an email. I am always open to new contacts, research problems and collaborations.
In particular, do get in touch if you are interested in doing a PhD in any of the described areas.
Main areas of my research
Waves in inhomogeneous media
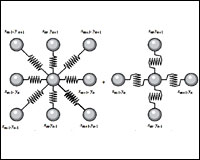 Asymptotic models of periodically inhomogeneous media; low- and high-frequency homogenisation;
boundary conditions for strain-gradient models
Asymptotic models of periodically inhomogeneous media; low- and high-frequency homogenisation;
boundary conditions for strain-gradient models
Localised vibrations and waves
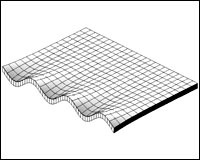 Propagation of stationary and non-stationary surface and edge waves; trapped modes in linear
elasticity; edge resonance phenomena
Propagation of stationary and non-stationary surface and edge waves; trapped modes in linear
elasticity; edge resonance phenomena
Structural optimisation
 Analytical solution of structural optimisation problems; optimality criteria; Michell
and Michell-Hemp structures, esp. featuring distributed loads
Analytical solution of structural optimisation problems; optimality criteria; Michell
and Michell-Hemp structures, esp. featuring distributed loads
Dynamics of pre-stressed structures
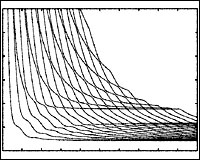 Small amplitude waves in finitely deformed solids; asymptotic models for low- and high-
frequency motion of thin pre-stressed plates
Small amplitude waves in finitely deformed solids; asymptotic models for low- and high-
frequency motion of thin pre-stressed plates
Non-stationary waves
 Elastic waves produced by instanteneous loads; far-field asymptotics for displacement fields;
the influence of geometric dispersion
Elastic waves produced by instanteneous loads; far-field asymptotics for displacement fields;
the influence of geometric dispersion
